circulation theorem
From Glossary of Meteorology
- V. Bjerknes's circulation theorem: 1) With reference to an absolute coordinate system, the rate of change of the absolute circulation dCa/dt of a closed individual fluid curve, that is, one that will consist always of the same fluid particles, is equal to the number of pressure–volume solenoids Nα,-p embraced by the curve
where the circulation has the same sense as the solenoids, the sense of the rotation from volume ascendent to pressure gradient.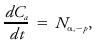
2) With reference to a relative coordinate system (specifically, the rotating earth), the rate of change of circulation relative to the earth dC/dt of an arbitrary closed individual fluid curve is determined by two effects: a) the solenoid effect that tends to change the circulation in the sense of the solenoids by an amount per unit time equal to the number of solenoids embraced by the curve; and b) the inertial effect that tends to decrease the circulation by an amount per unit time proportional to the rate at which the projected area of the curve in the equatorial plane expands:
where Ω is the angular speed of the earth's rotation and A is the equatorial projection of the curve. This is the most useful form of Bjerknes's circulation theorem. It permits the qualitative examination of many types of frictionless atmospheric motion that are too complicated for complete analytic treatment, for example, the sea breeze.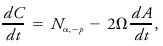
- Kelvin's circulation theorem: The rate of change of the circulation dC/dt of a closed individual fluid curve is equal to the circulation integral of the acceleration
 around the curve:
around the curve:
where dr is a vector line element of the curve.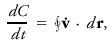
- Höiland's circulation theorem: An arbitrary closed tubular fluid filament with constant cross section has a total mass acceleration along itself equal to the resultant of the force of gravitation along the filament:
where ρ is the fluid density,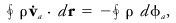
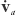 is the absolute vector acceleration, and dφa is the variation of the gravitational potential from the initial to the terminal point of the vector element dr. This theorem is particularly useful in the study of the stability of fluid flow.
is the absolute vector acceleration, and dφa is the variation of the gravitational potential from the initial to the terminal point of the vector element dr. This theorem is particularly useful in the study of the stability of fluid flow.
Gill, A. E. 1982. Atmosphere–Ocean Dynamics. Academic Press, . 226–231, 237–241.
Holton, J. R. 1992. An Introduction to Dynamic Meteorology. 3d edition, Academic Press, . 87–92.
