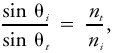refractive index
From Glossary of Meteorology
(Or index of refraction; also absolute refractive index.) The ratio of the free- space speed of light c, a universal constant, to the phase velocity of a plane harmonic electromagnetic wave in an optically homogeneous, unbounded medium.
The refractive index, often denoted as n, of a given material in a given state depends on the frequency of the plane wave. A plane harmonic wave incident on an optically smooth interface between two dissimilar, negligibly absorbing media undergoes a change in direction specified by Snel's law (attributed to Willebrord Snel, almost always misspelled as Snell):
Brillouin, L. 1960. Wave Propagation and Group Velocity.
Barr, E. S. 1955. Amer. J. Phys.. 623–624.
The refractive index, often denoted as n, of a given material in a given state depends on the frequency of the plane wave. A plane harmonic wave incident on an optically smooth interface between two dissimilar, negligibly absorbing media undergoes a change in direction specified by Snel's law (attributed to Willebrord Snel, almost always misspelled as Snell):
where θi is the angle between the normal to the interface and the wave vector of the (incident) wave in the medium with refractive index ni, and θt is the angle between the normal to the interface and the wave vector of the (transmitted or refracted) wave in the medium with refractive index nt. The ratio nt/ni is the relative refractive index. Strictly speaking, plane harmonic wave propagation in an unbounded, homogeneous medium is specified by a complex refractive index, sometimes written as
where n and k are nonnegative but otherwise unrestricted. The choice of sign depends on the convention for harmonic time dependence,
where ω is circular frequency. Here the real part n is as defined previously, and the imaginary part k, sometimes called the absorption index, is related to the absorption coefficient by
where λ is the free-space wavelength. The inverse of the absorption coefficient is the distance over which the irradiance of a plane wave is attenuated (by absorption) by the factor e. Although the real part of the complex refractive index is often denoted as n, no symbol is widely used for the imaginary part. In particular, the complex refractive index is sometimes written
in which instance the absorption coefficient is
where λ is the wavelength in the medium. Two widespread myths about n are that it can never be less than 1 and that it stands in a one-to-one relation with (mass) density. For a refutation of the first see Brillouin (1960); for a refutation of the second see Barr (1955).
Brillouin, L. 1960. Wave Propagation and Group Velocity.
Barr, E. S. 1955. Amer. J. Phys.. 623–624.